Have you ever wondered about the unique properties of a pentagon shape? This fascinating five-sided polygon holds secrets that are waiting to be unraveled. In this article, we will dive into the world of pentagons, exploring their characteristics, formulas, and even some intriguing examples. So, let's embark on this geometric adventure!
What is a Pentagon?
A pentagon is a two-dimensional shape with five sides and five angles. Derived from the Greek words "penta" meaning five and "gon" meaning angle, this shape captivates the imagination with its symmetrical allure. Feast your eyes on the pentagon shape itself:
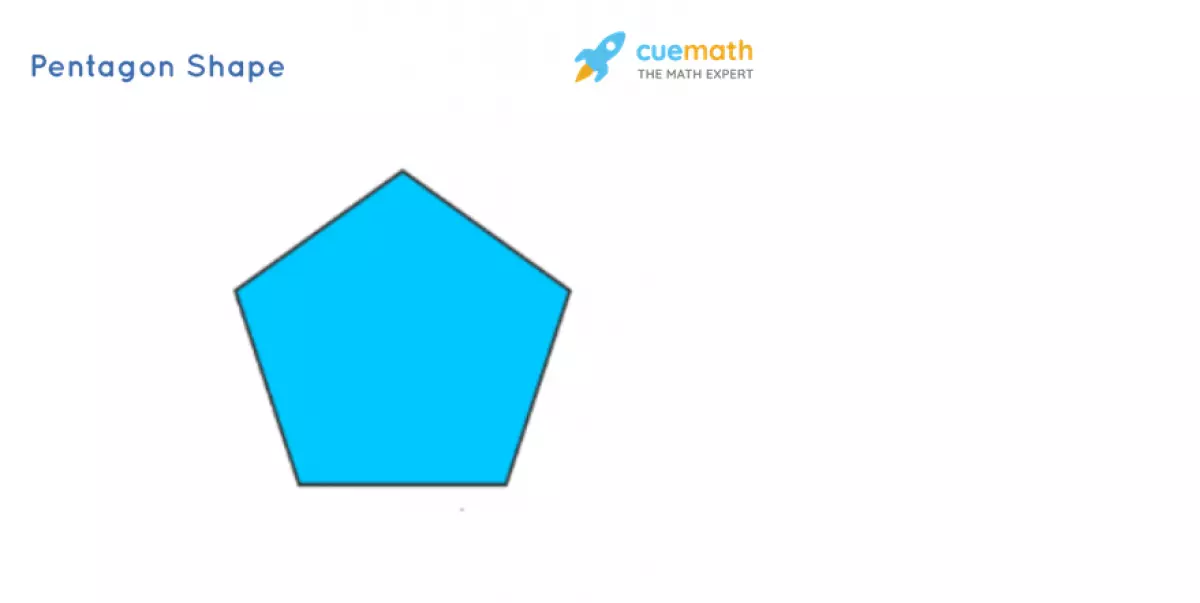Image: Pentagon shape
Pentagon Sides and Angles
As depicted in the image above, a pentagon boasts five sides and five interior angles. In the case of a regular pentagon, all sides are equal in length, and each interior angle measures 108°. However, irregular pentagons showcase angles of varying measures while still summing up to 540°.
Pentagon Properties
Let's delve into the fundamental properties of a pentagon, which will help us recognize this captivating shape:
- A pentagon possesses 5 sides and 5 angles.
- It allows for the creation of 5 diagonals, which can be calculated using the formula: Diagonals of a pentagon = n × (n − 3) ÷ 2 = 5 × (5 − 3) ÷ 2 = 5.
- The sum of all interior angles adds up to 540°, while the sum of the exterior angles totals 360°.
- In the case of a regular pentagon, each interior angle measures 108°, and each exterior angle measures 72°. These values can be calculated using the formulas: Interior angle of a regular pentagon = 540° ÷ n = 540° ÷ 5 = 108°, Exterior angle of a regular pentagon = 360° ÷ n = 360° ÷ 5 = 72°.
Pentagon Formulas
To further explore the mathematical intricacies of a pentagon, let's take a look at some essential formulas:
- Diagonals of a pentagon = n × (n − 3) ÷ 2 = 5 × (5 − 3) ÷ 2 = 5.
- Sum of interior angles of a pentagon = 180° × (n − 2) = 180° × (5 − 2) = 540°.
- Each exterior angle of a regular pentagon = 360° ÷ n = 360° ÷ 5 = 72°.
- Each interior angle of a regular pentagon = 540° ÷ n = 540° ÷ 5 = 108°.
- Area of a regular Pentagon = 1/2 × Perimeter × Apothem.
- Perimeter of Pentagon = (side 1 + side 2 + side 3 + side 4 + side 5).
Pentagon Shape Objects
The pentagon shape can be found in various objects we encounter in our daily lives. Take, for instance, the shape of an okra segment or the symmetrical beauty of a starfish. Below are a few captivating examples of regular and irregular pentagon shapes:
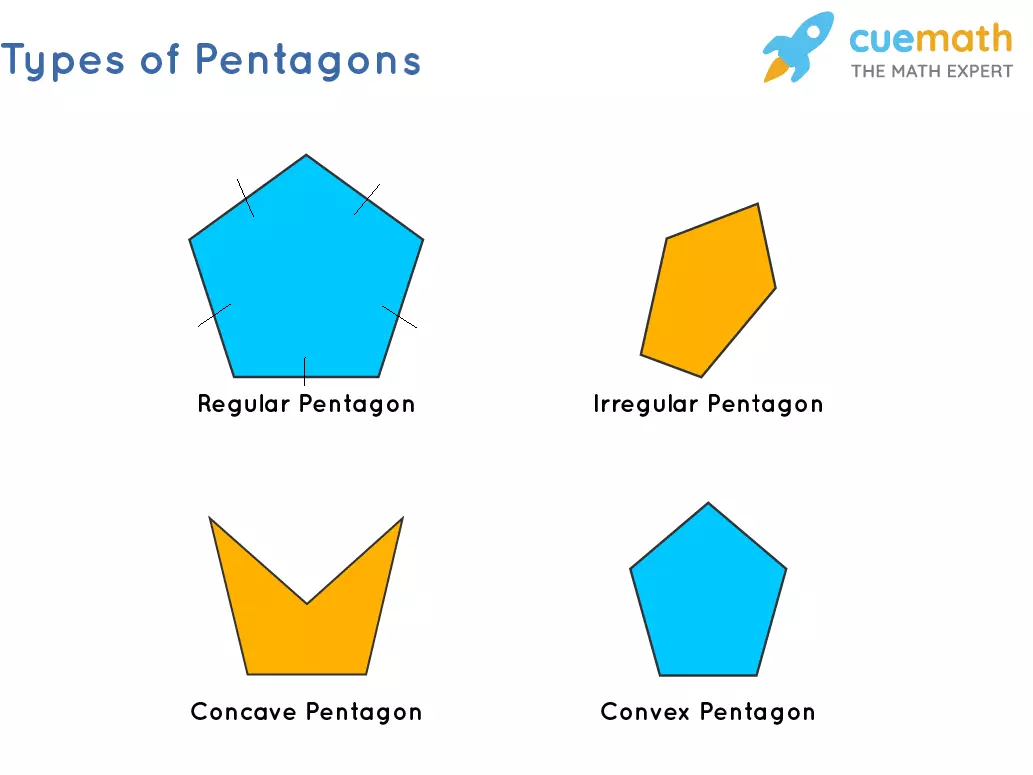Image: Pentagon Shape Examples
Interesting Facts about the Pentagon Shape
Did you know that the Pentagon is not only a geometric shape but also the headquarters of the United States Department of Defense in Washington DC? Let's explore some intriguing facts about this remarkable structure:
- The Pentagon was commissioned by President Roosevelt during World War II to serve as the new Department of War building.
- The architect chose the pentagon shape for its design, minimizing the distance individuals needed to traverse between offices, improving efficiency and accessibility.
Regular Pentagon and Irregular Pentagon
A pentagon can be classified as either a regular pentagon or an irregular pentagon based on its angle measures and side lengths:
- In a regular pentagon, all interior angles are equal in measure, and all sides are of the same length.
- In an irregular pentagon, both the interior angles and side lengths differ.
Convex Pentagon and Concave Pentagon
Furthermore, pentagons can be categorized as convex or concave depending on the orientation of their angles:
- A convex pentagon features interior angles that are all less than 180°, with its vertices pointing outwards.
- A concave pentagon, on the other hand, exhibits one or more interior angles exceeding 180°, and its vertices point inwards.
Image: Types of Pentagons
Conclusion
The pentagon shape captivates mathematicians and enthusiasts alike with its symmetrical elegance and intriguing properties. Whether it's exploring the formulas or discovering real-world examples, the pentagon shape never fails to amaze. So, the next time you encounter this captivating polygon, take a moment to appreciate its distinctive allure!
☛ Related Articles on Pentagon:
- Angles in a Pentagon
- Area of Pentagon
- Pentagon Area Calculator
- Decagon
- Pentagonal Pyramid
